B.
Sc. III Semester V
Subject-Statistics-XI
DSE-E15:
Sampling Theory
Theory:
36 Hours. (Credit 02)
Unit-1
Simple and Stratified Random
Sampling:
Introduction:
Sampling is quite often used in our day
to day practical life. For example - in
a shop we assess the quality of sugar, wheat or any other commodity by taking a handful of it
from the bag and then decide to purchase it or not. A housewife normally tests,
the cooked products to find if they are properly cooked and contain the proper
quantity of salt.
In sampling theory we first define the following
terms.
i)
Population (Universe)
The group of
individuals under study is called population or universe. (The totality of the objects of study) For example -if we are going to study the
economic conditions of primary teachers in Maharashtra state, then the total of
all the primary teachers in Maharashtra state is the population or universe for
the study. In short the totality of the members of study is called the
population. It may be a group of men, animals, trees or electric bulbs cars etc.
ii) Sample
A finite subset of
individuals in a population is called a sample and the number of individuals in
a sample is called the sample size. (A part of the population is called sample).
iii) Census method
The method of
collecting data from entire population is called the census method. If the census
method is to be followed in the above example then we have to collect data
about the economic conditions of every primary teacher in Maharashtra state.
iv) Sampling method
If instead of studying
the entire population, a part of it is studied it is called the sampling method.
Thus if the sampling method is to be used in the above example, we would study
the economic conditions of a few properly selected primary teachers and then
estimate the results for all the teachers. In short, if the data is collected
from a selected few it is called sampling method.
Advantages of sampling method over
census method
i) Time
If the population is large (generally it is),
then the study of the entire population not only for collection but also for
analyzing the data will require a lot of time. As against this collecting and
analysis of the sample will largely reduce the time required. In some cases
where the results are required quickly census method is not used
ii) Cost
It is also obvious that the study of entire
population will be very costly. Since in a sample survey only a part of is to
be studied, the cost involved will be proportionately less. Sampling method is much more economical than
the census method.
iii) Reliability (Accuracy)
Since in a sample, only
a part is to be studied a number of precautions can be taken and a very careful
investigation can be made. On the other hand information may be lost in census
method on account of the large size of the population. Due to small size of
sample, it is possible to check the information also to check the results
during analysis. All this leads to increased reliability of the sampling method.
iv) Details of
information
Again, since the size of the sample is small, every
member of the sample can be studied rigorously and detailed information can be
obtained about it.
v) In some cases sampling is the only
possible method
In certain investigations census method is not
possible to use and only the sampling method is used. For example: examining
blood of a human body, inspection of crackers, explosive materials, measuring
life time of electric components etc. In such cases sampling is the only
possible method. Thus sampling method is found to be much superior to the census
method.
Some concepts in sampling
Distinguishable Elementary Unit
The ultimate unit in population which
is distinguishable and identifiable is called as an elementary unit. For example; in population census survey every
individual person is an elementary unit.
The population under study must be
divided into small parts called sampling units or units. Sampling units
together must cover the entire population and they must not overlap. For
example: in a socio economic survey, a family is a sampling unit whereas in
health survey an individual will be sampling unit. In a population of light bulbs, the unit is a
single bulb. In sampling of agricultural crop the unit may be field or an area
of land whose size and shape is immaterial. Thus sampling unit is the smallest
part of the population which cannot be further subdivided for the said purpose.
Thus the sampling unit may consist of
one or more over elementary units of the population. A well defined and identifiable
elements or group of elements on which observations can be made is called
sampling units.
In order to cover the entire population, there
should be some list or map called the sampling frame. It is an exhaustive list
of all members or elements of population. It gives guidelines to cover the
entire population.
As the sampling frame
determines the structure of the sample survey, it must be up-to-date and non
overlapping. In a socio economic survey, frame may be determined from the
records at Gram panchayat or ration cards.
Samples can be selected in two ways.
Random sampling
In this method, the sample is selected
impartially. Personal or any kind of bias
in selection is avoided and pure statistical approach is used. These methods least
affected by personal bias, so these methods are widely used in practice. It is
also referred as probabilistic sampling; since it is random sampling laws of
probability can be applied.
Advantages
i) Random sampling does not need the detailed
information about the population for its effectiveness.
ii) It provides an estimate and has measurable precision.
iii) It is possible to evaluate the relative
efficiency of various sample designs only when random sampling is used.
Limitations
i) It requires a very high level of skills and
experience for its use.
ii) To plan and to execute a random sample, a lot of
time is required.
iii) As compare to non random sampling, the cost
involves in random sampling is large.
Due to these
limitations, non random sampling is used quite often in practice.
Non random sampling
It is a process of
sampling without randomization. A non random sample is selected on the basis of
judgment or convenience and not under the probability consideration. Investigators select elements in any manner
suitable to him. For example: he may select elements in first come first serve
basis.
To select candidates for debate competition,
deliberate selection of suitable candidates will be done. It is purposive
sampling (non random). In the advertisement campaign for cosmetics, certainly a
sample of youngsters will be taken. This method is unscientific and produces unreliable
results.
Convenient
Sampling:
It involves selecting participants who
are easily accessible and convenient to reach.
Example:
A researcher wants to study the average amount spent on coffee per day. They
stand outside a popular coffee shop and ask customers exiting the shop about
their daily coffee expenses. This sample is convenient, but may not represent
the entire population.
Purposive
Sampling:
It involves selecting participants based
on specific criteria or characteristics relevant to the research question.
Example:
A researcher wants to study the experiences of entrepreneurs who have
successfully launched startups. They select participants from a list of
award-winning entrepreneurs, ensuring that the sample has the desired expertise
and experience.
Judgment
Sampling:
It
involves selecting participants based on the researcher's expertise and
knowledge about the population.
Example:
A researcher wants to study the impact of a new teaching method on student
learning outcomes. They select classrooms and students based on their knowledge
of the schools and teachers, ensuring a representative sample of the
population.
Snowball
sampling:
It is used to select participants for a
study, particularly in cases where the population is hard to reach or hidden.
It's called "snowball" because the sample size grows incrementally,
like a snowball rolling down a hill, gathering more participants as it goes.
Example:
Studying a rare disease, researchers start with a few patients (seeds) and ask
them to refer other patients they know, creating a snowball effect to gather
more participants.
Quota sampling:
It is a non-probability sampling
technique used in research to select participants that represent specific
subgroups or characteristics of a population. In quota sampling, the population
is divided into subgroups based on relevant characteristics, such as age,
gender, income, occupation; etc. The researcher then sets a quota (a specific
number) for each subgroup, ensuring that the sample is representative of the
population's diversity. Participants are selected based on these quotas, often
through convenience or snowball sampling methods.
Quota
sampling is commonly used in market research, social sciences, and opinion
polls, where the goal is to understand specific segments of the population
rather than the entire population.
Note:
1.
As sample is selected to study the population, it should be such that it will
represent all important characteristics of the population. Thus sample is miniature
of population.
2.
Sampling units should be independent.
3.
It should be evenly spread over the population. It can be achieved by dividing
population in homogeneous subgroups and selecting samples from each subgroup.
Methods of sampling
There are various methods used to select
the sample from the population. We shall study the following method of sampling.
Simple random sampling (SRS)
In this method, each item in the
population has an equal and independent chance of being selected in the sample.
Suppose we take a
sample of size n from a finite population of size N, then there are NCn 
possible samples. A sampling method in
which each of the NCn samples has an equal chance of
being selected is known as random sampling and the sample obtained by this
method is called as a random sample. The following methods are commonly used
for selecting a simple random sample.
Lottery method
In this method, the numbers or the names
of all the members of the population are written on separate pieces of paper of
the same size, shape and color. The
pieces are folded in the same manner, mixed up thoroughly in a drum and the
required numbers of pieces are drawn blindly. All this ensures that, each member of the
population has equal opportunity of being included in the sample. The method is
used for drawing the prizes of a lottery and hence the name.
Table of random numbers
If population is large, lottery method
is tedious to follow. An alternative
method is the method of random numbers. In this method, all the items are given
numbers. Then a book of random numbers is taken. The book is opened at random
and from any row any column, the numbers are taken. The items bearing these
numbers are included in the sample.
SRSWR and SRSWOR
If the units are selected one by
one in such a way that, a unit selected is replaced back to the population before the next draw
(selection), it is known as SRSWR. If a unit selected once is not replaced back
to the population before the next draws (selections), it is known as SRSWOR.
For ex.: Population of
size N= 4, contains say 1, 2, 3 & 4
items, then the SRSWR and SRSWOR’s
of size 2 are,
SRSWOR SRSWR
(1, 2), (1, 3), (1, 4) (1,2), (1,3), (1,4 ),(1,1), (2,3), (2,4),
(2,3), (2,4) (2,2),(3,4),(3,3),
(3, 4) (3,2), (4, 1), (4,2), (4,3)
Total = 06 Total = 16
***
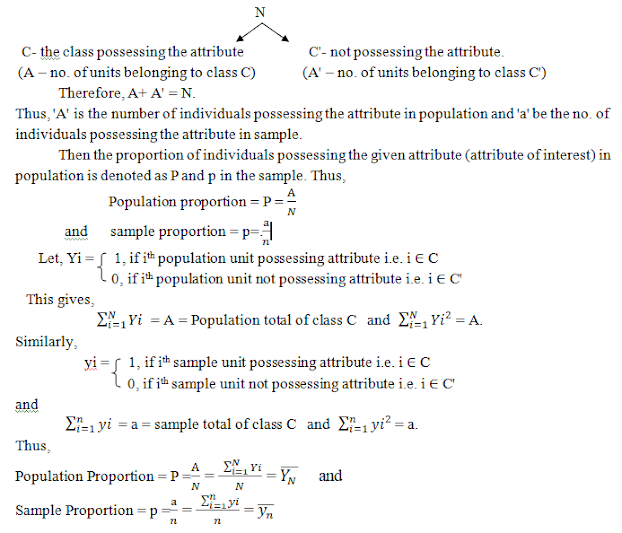
Sampling of dichotomous attributes
Population
may be divided into two or more classes according to attributes. An attribute
which can be classified into two classes is called as dichotomous attribute and
the classification is called dichotomous classification.
For
ex: If attribute is gender then population is classified into two classes male
and female.
Determination of sample size
In sampling theory, some of the most important
problems for statisticians or researchers may face before planning for the
sample survey are:
I) What should be the size of sample?
II) How large or small should be the sample that it
may be representative of the whole population?
III) Whether the estimated sample consists of the
smallest sampling error?
IV) How to determine the sample size for further
statistical study?
Two important facts are considered at the time of determining
sample as,
I)
If sample size is too small, it may not serve to achieve the objective of the
study.
II)
If sample size is too large, it may require huge money (cost of study), time
and human resources.
I)
When margin of error (d) & confidence coefficient (1-α) is known (pre specified).
II)
When coefficient of variation (C.V.) and confidence coefficient (1-α) is known.
Comments
Post a Comment